Sampling techniques for rare events

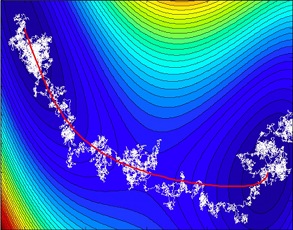
Dynamical systems are often subject to random perturbations or noise. Even when the noise amplitude is very small, it has a profound influence on the dynamics on the appropriate time-scale. At a first sight one might think that this influence happens at such a long time-scale that it is rarely of practical importance. That this is an incomplete view may be understood by noticing that most physical processes will not happen at zero temperature when thermal noise is absent. In reality nature presents one with a very wide range of temporal scales. The small noise induces events which are rare with respect to the internal clock of the system, but this clock can be very fast, and the processes of interest to our daily lives are mostly on the order of seconds or longer. This leaves plenty of room for rare events caused by thermal noise to make their appearance. In fact, phenomena like nucleation events during phase transitions, chemical reactions, conformation changes of biomolecules, bitable behaviors in genetic switches, or regime changes in climate are just a few examples of rare events among many others.

Traditionally the methods of choice for a quantitative understanding of the effect of noise has been Monte Carlo or direct simulation of Langevin equations. When the noise is small, which is the case of interest here, these methods become prohibitively expensive, due to the presence of two disparate time-scales: the time-scale of the deterministic dynamics and the time-scale between the rare events caused by the noise. Noticing this difficulty, alternative theories and numerical methods have been proposed.

This section contains the works where we developed theoretical tools and their numerical counterparts to identify the pathways of rare events in complex systems and estimate their rate of occurrence and associated free energy. These works include some reviews; papers on transition path theory (TPT), a new theoretical framework to go beyond large deviations theory (LDT) in situations where entropic effects dominate; papers on the string method and the minimum action method, which are numerical techniques based on LDT and TPT to identify the transition pathways of rare events in complex systems; papers on transition state theory, in which an original viewpoint is taken on this well-known theory; and finally papers on various sampling techniques for the Boltzmann-Gibbs probability distribution with or without constraints, or its marginal in a few collective variables to compute the free energy associated with these variables.






Transition Path Theory Transition path theory (TPT) is a theoretical framework to describe the statistical properties of reactive trajectories, that is, the pieces of a long ergodic trajectory during which this trajectory hops from a given set A in configuration space to a given set B. TPT gives the probability distribution of the reactive trajectories, their probability current and their rate. TPT also provides the theoretical background for the string method.
String method and applications The string method is a simple and efficient algorithm to move curves over an energy landscape. At zero temperature, it identifies the minimum energy path (MEP); at finite temperature, it identifies the streamline of the probability current of reactive trajectories which carries most of the probability flux of these trajectories (and reduces to the MEP in the zero temperature limit). Both the zero-temperature and the finite-temperature string methods can be applied either in Cartesian space or using collective variables; in the latter case, the string method allows one to identify minimum free energy paths (MFEPs).
Minimum action method and applications The minimum action method is a numerical technique to identify the minimizers of the Freidlin-Wentzell large deviations action functional. In the small noise limit, these minimizers are the paths of maximum likelihood by which various events occur like, e.g. the switching from one metastable basin to another. The minimum action method applies for gradient and nongradient systems as well as for Markov jump processes arising e.g. in the context of chemical kinetics and genetic switches.
Transition state theory and generalizations Transition state theory (TST), originally developed in the eraly 19th century by Marcelin, Eyring, Wigner and Horiuti gives an exact expression for the mean frequency of transition between any two sets partitioning the system phase-space. TST also gives the mean residency times in each of these sets. In the papers below, we take an original viewpoint on the theory and show that TST, suitably modified, can give the exact mean frequency between any two sets in the system phase-space, like e.g. metastable sets, whether or not these sets partition the state-space. This generalization is a slight modification on the standard way dynamical corrections are accounted for, and it allows to derive precise statistical error estimates on the numerical predictions of the theory. We also show how to optimize upon the dividing surface to minimize these errors.
Free energy calculations, sampling methods, etc. The techniques discussed here usually require one to sample the Botzmann-Gibbs probability distribution, with or without hard constraints, or the marginal of this distribution in some collective variables to compute their free energy. In the papers below, several numerical techniques to perform these operations are introduced.